A matrix that captures the evolution of the age distribution of the females in a population. The female population is divided into g age-groups, each of width k years. The probability that a female, in group j at time t, will be alive in group (j+1) at time (t+k) is denoted by Sj. The mean number of female offspring born to a mother in group j between times t and (t+k), and alive at time (t+k), is denoted by Mj. The Leslie matrix, L, is given by
 The entries in this matrix are the mean numbers at time (t+k) resulting from single individuals in each group at time t. If N(t) is the g×1 column vector with entries being the mean numbers of females in the groups at time t then the Leslie model states that
The entries in this matrix are the mean numbers at time (t+k) resulting from single individuals in each group at time t. If N(t) is the g×1 column vector with entries being the mean numbers of females in the groups at time t then the Leslie model states that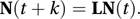
- external node
- external path length((of a tree))
- external relations
- external schema((of a database))
- external sorting
- external storage
- external world
- exteroceptor
- extinction
- extinction, atmospheric
- extinction coefficient
- extinction, interstellar
- extinguishing agent
- extra-binomial variation
- extracellular
- extracellular matrix
- extraclast
- extract
- extraction
- extractive distillation
- extractor
- extraembryonic membranes
- extraformational
- extrafusal
- extragalactic nebula