A theorem, given by Cochran in 1934, concerning sums of chi-squared variables. Let Y be an n×1 vector of independent standard normal variables (see normal distribution) and let A1, A2,…, Ak be non-zero symmetric matrices such that
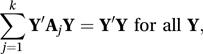 where Y′ is the transpose of Y. Write Qj=Y′AjY. Cochran's theorem, published in 1934, states that, if any one of the following three conditions is true, then so are the other two:
where Y′ is the transpose of Y. Write Qj=Y′AjY. Cochran's theorem, published in 1934, states that, if any one of the following three conditions is true, then so are the other two:(i) The ranks of A1, A2,…, Ak sum to n.
(ii) Each of Q1, Q2,…, Qk has a chi-squared distribution.
(iii) Each of Q1, Q2,…, Qk is independent of all the others.
- muscarinic
- Muschelkalk
- Musci
- muscle
- muscle scar
- muscle spindle
- muscovite
- muscularis mucosae
- MUSES
- Muses-C
- Museveni, Yoweri Kaguta
- Musgrave, Story (1935– )
- mush area
- Musharraf, Pervez (1943–)
- mushroom gate
- mushroom rock
- music
- Musical Instrument Digital Interface
- musical instrument digital interface
- musical scale
- music of the spheres
- musketeer
- Muskogee
- musk ox
- muskrat