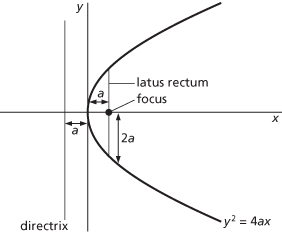
A conic with eccentricity equal to 1. Thus a parabola is the locus of all points P such that the distance from P to a fixed point F (the focus) is equal to the distance from P to a fixed line l (the directrix). It is obtained as a plane section of a cone in the case when the plane is parallel to a generator of the cone (see conic). A line through the focus perpendicular to the directrix is the axis of the parabola, and the point where the axis cuts the parabola is the vertex. It is possible to take the vertex as origin, the axis of the parabola as the x-axis and the focus as the point (a,0). In this coordinate system, the directrix has equation x =−a and the parabola has equation y² =4ax (see normal form of conics).

A parabola with focus and directrix

Different values of a give parabolas of different sizes, but all parabolas are the same shape (i.e. are similar to one another). The equations x =  at², y =  2at are parametric equations for the parabola y² = 4ax.

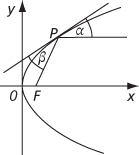
For a point P on the parabola, let α‎ be the angle between the tangent at P and a line through P parallel to the axis of the parabola, and let β‎ be the angle between the tangent at P and a line through P and the focus, as shown in the figure below;

A parabolic reflector

then α‎ = β‎. This is the basis of the parabolic reflector: if a source of light is placed at the focus of a parabolic reflector, each ray of light is reflected parallel to the axis, so producing a parallel beam of light.

http://www.calculus.org/Contributions/animations.html Properties of the parabola with links to animated illustrations.