A line that a curve approaches but only touches at infinity.
A line l is an asymptote to a curve if the distance from a point P to the line l tends to zero as P tends to infinity along some unbounded part of the curve. Consider the following examples:
Example (i) has x =−2 and x =1 as vertical asymptotes and y = 0 as a horizontal asymptote. Example (ii) has no vertical asymptotes and y = 3 as a horizontal asymptote. To investigate example (iii), it can be rewritten as
Then it can be seen that y = x−1 is a slant (or oblique) asymptote, that is, an asymptote that is neither vertical nor horizontal.
If part of a graph is unbounded and there is a fixed straight line l such that the distance from a point P on the graph to l tends to 0 as OP → ∞, where O is the origin, then l is an asymptote to the curve. Alternatively, it is the limiting position of the tangent to the graph at P, as OP → ∞. For example, the asymptotes of the graph of
 are y=2x+3 and x=2.
are y=2x+3 and x=2.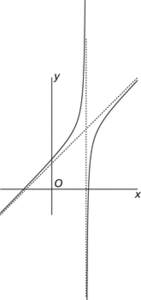
Asymptote. The dotted lines are the asymptotes (vertical, x=2; oblique, y=2x+3) of the graph y=2x+3−.
A straight line that is closely approached by a curve so that the perpendicular distance between them decreases to zero at an infinite distance from the origin.
- Neohelikian
- neoichnology
- Neo-Kantianism
- neo-Lamarckism
- neoliberalism
- Neolithic
- neomineralization
- neomorphism
- neon
- neonicotinoid
- neon lamp
- neon(symbol: Ne)
- neopatrimonialism
- neoplasm
- Neoplatonism
- neoprene
- Neoproterozoic
- Neo-Pythagoreanism
- Neornithes
- neostratotype
- Neo-Taoism
- neotectonics
- neoteny
- Neotethys
- Neo-Thomism