A remarkable result presented by Stein in 1956. Suppose n vector observations {xj} are taken from a multivariate normal distribution with p (> 3) dimensions and unknown mean μ. The most efficient estimator of μ is not x̄, the sample mean, but is
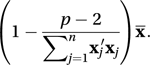The estimators of this type presented by Willard James and Stein in 1961 are called James–Stein estimators or shrinkage estimators (since they ‘shrink’ x̄ towards the zero vector).