Events A and B are independent if the occurrence of either does not affect the probability of the other occurring. Otherwise, A and B are dependent. For independent events, the probability that they both occur is given by the product law Pr(A ∩ B) = Pr(A) Pr(B). This is equivalent to the conditional probability Pr(A | B) equalling Pr(A), provided Pr(B)≠0.
For example, when an unbiased coin is tossed twice, the probability of obtaining ‘heads’ on the first toss is , and the probability of obtaining ‘heads’ on the second toss is . These two events are independent. So the probability of obtaining ‘heads’ on both tosses is equal to .
Two events (see sample space) A and B are independent events if
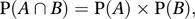
or, equivalently, if P(A|B) = P(A), or if P(B|A) = P(B). Three events A, B, and C are said to be independent (or mutually independent) if each pair is independent and if, in addition,
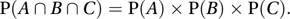 For a set of more than three events to be independent the multiplication law for probabilities must hold for all possible subsets. See also conditional probability; intersection; pairwise independent.
For a set of more than three events to be independent the multiplication law for probabilities must hold for all possible subsets. See also conditional probability; intersection; pairwise independent.
- Lawes, Sir John Bennet
- Law, John (1671–1729)
- lawlike
- lawnmower
- law of averages
- law of chemical equilibrium
- law of compound probability
- law of conservation
- law of conservation of energy
- law of conservation of mass
- law of constancy of interfacial angles
- law of constant composition
- law of constant proportions
- law of correlation of facies
- law of cross-cutting relationships
- law of definite proportions
- law of demand
- law of diminishing returns
- law of effect
- law of excluded middle
- law of faunal succession
- law of Haüy
- Law of Independent Assortment
- law of large numbers
- law of mass action