Although various laws concerning lines and angles were known to the Egyptians and the Pythagoreans, the systematic treatment of geometry by the axiomatic method began with the Elements of Euclid. From a small number of explicit axioms, postulates, and definitions Euclid deduces theorems concerning the various figures of geometrical interest. Until the 19th century this work stood as a supreme example of the exercise of reason, which all other intellectual achievements ought to take as a model. With increasing standards of formal rigour it was recognized that Euclid does contain gaps, but fully formalized versions of his geometry have been provided. For example, in the axiomatization of David Hilbert, there are six primitive terms, in that of E. V. Huntington only two: ‘sphere’ and ‘includes’.

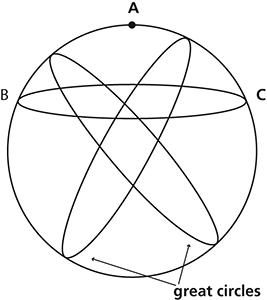
In the work of Kant, Euclidean geometry stands as the supreme example of a synthetic a priori construction, representing the way the mind has to think about space, because of the mind’s own intrinsic structure. However, only shortly after Kant was writing non-Euclidean geometries were contemplated. They were foreshadowed by the mathematician K. F. Gauss (1777–1855), but the first serious non-Euclidean geometry is usually attributed to the Russian mathematician N. I. Lobachevsky, writing in the 1820s. Euclid’s fifth axiom, the axiom of parallels, states that through any point not falling on a straight line, one straight line can be drawn that does not intersect the first. In Lobachevsky’s geometry several such lines can exist. Later G. F. B. Riemann (1822–66) realized that the two-dimensional geometry that would be hit upon by persons confined to the surface of a sphere would be different from that of persons living on a plane: for example, π‎ would be smaller, since the diameter of a circle, as drawn on a sphere, is relatively large compared to the circumference. In the figure, bcB, the circumference of the circle, is less than 2π‎AB, where AB is the radius. Generalizing, Riemann reached the idea of a geometry in which there are no straight lines that do not intersect a given straight line, just as on a sphere all great circles (the shortest distance between two points) intersect.

The way then lay open to separating the question of the mathematical nature of a purely formal geometry from the question of its physical application. In 1854 Riemann showed that space of any curvature could be described by a set of numbers known as its metric tensor. For example, ten numbers suffice to describe the point of any four-dimensional manifold. To apply a geometry means finding coordinative definitions correlating the notions of the geometry, notably those of a straight line and an equal distance, with physical phenomena such as the path of a light ray, or the size of a rod at different times and places. The status of these definitions has been controversial, with some such as Poincaré seeing them simply as conventions, and others seeing them as important empirical truths. With the general rise of holism in the philosophy of science the question of status has abated a little, it being recognized simply that the co-ordination plays a fundamental role in physical science. See also relativity theory, space-time.