The extension of the method of least squares procedure to the case where the observations have been taken on random variables that are not all independent of one another. The GLS estimate of the (p + 1)×1 parameter vector β in the multiple regression model

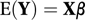
is given by

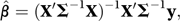
where y is an n×1 column vector of observations, Σ⁻¹ is the inverse of the variance-covariance matrix, and, with p explanatory variables and a constant term in the model, X is the n×(p+1) design matrix (see multiple regression model), and X′ is the transpose of X. See also weighted least squares.