An alternative to the Kolmogorov–Smirnov test for testing the hypothesis that a set of data come from a specified continuous distribution. The test was suggested independently by Cramér in 1928 and von Mises in 1931. The test statistic W (sometimes written as W2) is formally defined by
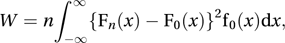 where F0(x) is the distribution function specified by the null hypothesis, Fn(x) is the sample distribution function, and f0(x)=F0′(x). In practice the statistic is calculated using
where F0(x) is the distribution function specified by the null hypothesis, Fn(x) is the sample distribution function, and f0(x)=F0′(x). In practice the statistic is calculated using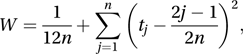 where
where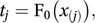 and x(j) is the jth ordered observation (x(1)≤x(2)≤⋯≤x(n)).
and x(j) is the jth ordered observation (x(1)≤x(2)≤⋯≤x(n)).The test has been adapted for use with discrete random variables, for cases where parameters have to be estimated from the data, and for comparing two samples. A modification leads to the Anderson–Darling test.
- Luddite
- Topa Inca
- topaz
- top carnivore
- top-down development
- top-down hierarchical design
- top-down nanofabrication
- top-down parsing
- tope
- TOPEX/Poseidon
- topic drift
- topic-neutral
- toplap
- top-level domain
- topocentric
- topocentric coordinates
- top of atmosphere
- topographic correction
- topography
- topoisomer
- topoisomerase
- topological band theory
- topological close packing
- topological data analysis
- topological defect