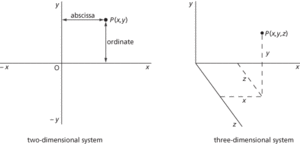
A system used in analytical geometry to locate a point P, with reference to two or three axes (see graph). In a two-dimensional system the vertical axis is the y-axis and the horizontal axis is the x-axis. The point at which they intersect is called the origin, O. Values of y <0 fall on the y-axis below the origin, values of x<0 fall on the x-axis to the left of the origin. Any point P is located by its perpendicular distances from the two axes. The distance from the x-axis is called the ordinate; the distance from the y-axis is the abscissa. The position is indicated numerically by enclosing the values of the abscissa and the ordinate in parentheses and separating the two by means of a comma, e.g. (x,y). In three dimensions the system can be used to locate a point with reference to a third, z-axis. The system is named after the French mathematician René Descartes (1596–1650).
The method of Cartesian coordinates was very important in the history of mathematics and its physical applications, because it enabled methods from algebra to be used to solve geometrical problems and vice versa.
Cartesian coordinates.
See coordinates (in Euclidean space).
A coordinate system in which the position of a point or object is given with reference to either two or three planes at right angles to each other. For a two-dimensional system, the planes are represented by horizontal and vertical axes labelled x and y respectively. For a three-dimensional system, as used for example when describing the position of a planet, a third axis is introduced, labelled z. The system was introduced by the French mathematician René Descartes (1596–1650).
The usual system for identifying the location of a point in two, or more, dimensions. The position of a point P in a plane can be represented by a pair of numbers (x, y), relative to two axes Ox and Oy which are straight lines meeting at the origin O represented by (0, 0). In the usual case of rectangular (or perpendicular, or orthogonal) axes, the abscissa x is the perpendicular distance of P from Oy and the ordinate y is the perpendicular distance of P from Ox. The value of x is positive if P lies in the half-plane to the right of O, and is negative in the left-hand half-plane. Similarly, the value of y is positive in the upper half-plane and negative in the lower half-plane. The plane is therefore divided into four quadrants corresponding to the four combinations of coordinate signs. The units of distance in the directions Ox and Oy may be different and are usually indicated by numbers on the axes.
The term ‘Cartesian’ is derived from Descartes who first introduced coordinates. The word ‘coordinate’ was introduced by Leibniz in about 1693. The phrase ‘Cartesian coordinates’ was used in 1844.
The idea of coordinates can be generalized to three-dimensional space. The process can be reversed by considering any ordered set of three real numbers (x, y, z) as a point in three-dimensional space. This can be further generalized by considering the row vector (x1 x2…xn) to be the coordinates of a point in an n-dimensional space. This space is usually denoted by ℝn.
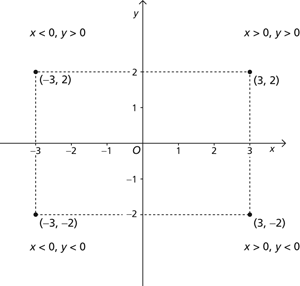
Cartesian coordinates. The axes meet at the origin O. The coordinates of a point are (x, y), where x and y are the corresponding signed distances along the two axes.
- anaphora
- anaphoresis
- Konfrontasi
- Kongo kingdom
- Konoe Fumimaro (1891–1945)
- kookaburra
- Koopmans, Tjalling Charles (1910–85)
- Koopmans’ theorem
- kopje
- koppie
- Kopp’s rule
- Koptev, Yuri Nikolayevich (1940– )
- Koran
- Korangan
- Korea
- Korea Advanced Institute of Science and Technology
- Korea Aerospace Research Institute
- Korea Multi-Purpose Satellite
- Korean Committee of Space Technology
- Korea, North
- Korean VLBI Network
- Korean War (1950–53)
- Korea, South
- Korea Space Launch Vehicle
- Kornberg, Arthur