A square symmetric matrix in which the elements on the main diagonal are variances and the remaining elements are covariances. Suppose X1, X2,…, Xp are random variables and the variance of Xj is and the covariance of Xj and Xk is cjk=ckj. Then the variance–covariance matrix is Σ, given by
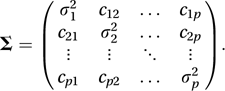 The same term is also used for the corresponding matrix based on sample values. If the matrix is diagonal then the variables display orthogonality.
The same term is also used for the corresponding matrix based on sample values. If the matrix is diagonal then the variables display orthogonality.
See covariance matrix.
- potential reserve
- potential temperature
- potential transformer
- potentiation
- potentiometer
- potentiometric surface
- potentiometric titration
- pot-hole
- pothole
- potlatch
- potometer
- POTS
- Potsdam Conference (17 July–2 August 1945)
- Potsdam gravity
- pot still
- Potter, Beatrice
- Potter’s flood-peak formula
- potto
- Potts model
- poultry
- pound
- poundal
- Poundian
- Pourbaix diagram
- pour point