A well-known, but intriguing probability problem. There are n people in a room. Assume that none is born on 29 February and that the remaining 365 days are all equally likely as birthdays. What is the smallest value of n for which the probability that at least two have the same birthday is greater than 0.5?
The answer is not 183, but 23. The complementary event is that all n people have different birthdays. The probability of this, pn, is
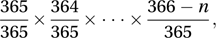which reduces surprisingly quickly as n increases:n
3
5
9
13
16
19
22
23
26
30
34
40
46
pn
0.99
0.97
0.91
0.81
0.72
0.62
0.52
0.49
0.40
0.29
0.20
0.11
0.05
- bolometer
- bolometric correction
- bolometric magnitude
- Bolshevik
- bolson
- Boltwood, Bertram Borden
- Boltwood, Bertram Borden (1870–1927)
- Boltzmann constant
- Boltzmann constant(symbol: k)
- Boltzmann equation
- Boltzmann formula
- Boltzmann, Ludwig (1844–1906)
- Boltzmann, Ludwig Eduard (1844–1906)
- Boltzmann, Ludwig Edward
- bolus
- Bolyai, Janos
- Bolyai, János (1802–60)
- Bolzano, Bernard (1781–1848)
- Bolzano-Weierstrass Theorem
- Bolzano’s Theorem
- Bolívar, Simón (1783–1830)
- BOM
- bomb
- Bombay Stock Exchange
- bomb calorimeter