A stronger version of the Chebyshev inequality. Let X1, X2,…, Xn be independent random variables, such that, for j=1, 2,…, n, Xj has expected value 0 and | Xj |≤M. Bernstein showed, in a 1926 paper, that, for all positive ε,
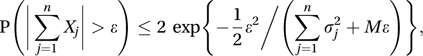where Var(Xj) = σj2. See also Chebyshev inequality; Hölder inequality; Kolmogorov inequality; Markov inequality; Minkowski inequality.
- pyroelectricity
- pyrogallol
- pyrogenetic minerals
- pyrolite model
- pyrolusite
- pyrolysis
- pyrometasomatic deposit
- pyrometer
- pyrometric cones
- pyrometry
- pyromorphite
- pyrones
- pyrope
- pyrophoric
- pyrophoricity
- pyrophosphoric acid
- pyrophyllite
- pyrosilicate
- pyrosulphuric acid
- pyrotechnic device
- pyroxene
- pyroxene gneiss
- pyroxene hornfels facies
- pyroxenes
- pyroxenite