If Y1 has a non-central chi-squared distribution with ν1 degrees of freedom and non-centrality parameter λ, and if Y2 is independent of Y1 and has a chi-squared distribution with ν2 degrees of freedom, then the ratio X, where
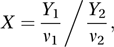 is said to have a non-central F-distribution with non-centrality parameter λ and with ν1 and ν2 degrees of freedom. The probability density function f of X is given by
is said to have a non-central F-distribution with non-centrality parameter λ and with ν1 and ν2 degrees of freedom. The probability density function f of X is given by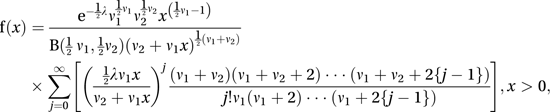 where B is the beta function. The distribution has mean and variance given by
where B is the beta function. The distribution has mean and variance given by respectively (with ν2>4). The distribution is unimodal.
respectively (with ν2>4). The distribution is unimodal.
- geological barrier
- geological column
- Geological Long Range Inclined Asdic
- Geological Society of America
- Geological Society of London
- geological time
- geological time scale
- geologic cross-section
- geologic map
- geologic map symbols
- geologic timescale
- geologic-time unit
- geology
- geomagnetic dipole field
- geomagnetic equator
- geomagnetic field
- geomagnetic polarity interval
- geomagnetic pole
- geomagnetic reversal timescale
- geomagnetic storm
- geomagnetism
- geomatics
- geometrical albedo
- geometrical isomerism
- geometrical libration