If Z1, Z2,…, Zν are independent standard normal variables (see normal distribution) and δ1, δ2,…, δν are constants then X, given by
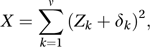 is said to have a non-central chi-squared distribution with ν degrees of freedom and non-centrality parameter λ, where
is said to have a non-central chi-squared distribution with ν degrees of freedom and non-centrality parameter λ, where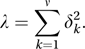 The probability density function f of X is given by
The probability density function f of X is given by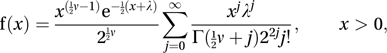 where Γ is the gamma function. The distribution, which is unimodal, has mean (ν+λ) and variance 2(ν+2λ). If λ=0 then the distribution becomes the chi-squared distribution with ν degrees of freedom.
where Γ is the gamma function. The distribution, which is unimodal, has mean (ν+λ) and variance 2(ν+2λ). If λ=0 then the distribution becomes the chi-squared distribution with ν degrees of freedom.
- spintronics
- spin wave
- spiny sharks
- spin–lattice relaxation
- spin–orbit coupling
- spin–statistics theorem
- spiracle
- spiral
- spiral arm
- spiral galaxy
- spiral inductor
- spiral model
- spiral scanning
- spire
- Spiriferida
- spiriferids
- spirillum
- Spirit
- spirit
- spirits of salt
- spiritualism
- spirochaete
- spiro compound
- spirometer
- spissatus