Let m2 = E((X−μ)2) and m4 = E((X−μ)4), where μ = E(X), be the second and fourth moments about the mean. Then is the kurtosis of the distribution. For a normal distribution this has the value 3, which can be taken as a standard for comparison and hence a value of 3 is termed mesokurtic—from ‘meso’ meaning ‘middle’. For values less than 3 the distribution is termed platykurtic, and for values greater than 3 it is leptokurtic.
A measure of the peakedness of a distribution. The measure (usually denoted by β2 or κ), was introduced by Karl Pearson before 1905. The measure is given by
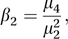
where μ4 is the fourth central moment of the distribution and μ2 is the variance. It is invariant under a change of scale or origin.
An alternative definition uses (β2−3) to give a value of 0 for the normal distribution. With this definition, a distribution having negative kurtosis is described as being platykurtic (flatter), and a distribution having positive kurtosis is described as being leptokurtic (more peaked). A distribution having kurtosis zero is described as mesokurtic.
The degree of peakedness in a distribution curve. If kurtosis (K) is 1, the curve is said to be mesokurtic; if K is greater than 1 it is leptokurtic; if K is less than 1 it is platykurtic.
A measure of how ‘humped’ a probability distribution is, compared to a normal distribution with the same mean and variance. The kurtosis of a variable x with mean μ is defined by K = E [(x − μ)4]/[E(x − μ)2]2. A variable with a normal distribution has K = 3. A distribution with K > 3 is slim and long-tailed, with more weight in its centre and extremes and less at medium distances from the mean than a normal distribution. A distribution with K < 3 is fat and short-tailed, with less weight in its centre and extremes and more at medium distances from the mean than a normal distribution.
- radiating
- radiation
- radiation belt
- radiation belts
- Radiation Belt Storm Probes
- radiation budget
- radiation counter
- radiation damage
- radiation densimeter
- radiation efficiency
- radiation era
- radiation fog
- radiation inversion
- radiation laws
- radiationless decay
- radiation night
- radiation noise
- radiation, nuclear
- radiation pattern
- radiation potential
- radiation pressure
- radiation resistance
- radiation sickness
- radiation temperature
- radiation tracks