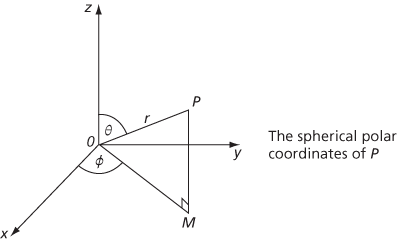
See polar coordinates.
Suppose that three mutually perpendicular directed lines Ox, Oy, and Oz, intersecting at the point O, and forming a right-handed system are taken as coordinate axes. For any point P, let M be the projection of P on the xy-plane. Let r = |OP|, let θ be the angle ∠zOP in radians (0 ≤ θ ≤ π) and let ϕ be the angle ∠xOM in radians (0 ≤ ϕ < 2π). Then (r, θ, π) are the spherical polar coordinates of P. (The point O gives no value for θ or ϕ, but is simply said to correspond to r = 0.) The Cartesian coordinates (x,y,z) of P in terms of r, θ, and ϕ are given by
Compare cylindrical polar coordinates.
Coordinates that specify the location of a point in three dimensions. The basis of these coordinates is a set of three mutually perpendicular axes, Ox, Oy, and Oz, that intersect at O. Consider a point P, in three-dimensional space, at a distance r from O, the angle being equal to θ. Let OM be the projection of OP on the xy-plane and let the angle be equal to ϕ. The spherical polar coordinates of P are (r, θ, ϕ), where 0° ≤ θ ≤ 180°, 0° ≤ ϕ < 360°. These coordinates are related to the alternative Cartesian coordinates (x, y, z) by
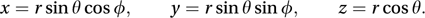
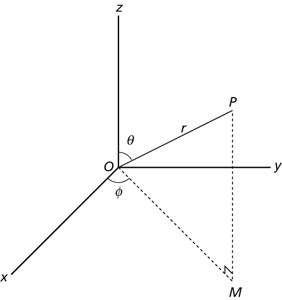
Spherical polar coordinates. Using Cartesian coordinates, the point P would be represented by (x, y, z), with x=r sin θ cos ϕ, y= r sin θ sin ϕ, z=r cos θ.
A technique for giving three-dimensional coordinates of a point based on its distance from a fixed origin and two angles relative to two perpendicular axes through that origin. The radial distance coordinate r can be interpreted as a move up the vertical z-axis, which is then followed by a rotation by polar angle θ down towards the horizontal x-axis, and finally a rotation by the azimuth angle φ about the z-axis towards the y-axis. There is no fixed convention as to the Greek symbols used for the two angles, so care is needed when using them. See also cylindrical polar coordinates; polar coordinates.
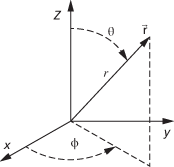
Spherical polar coordinates
- kinetochore
- kinetoplast
- Kinetoplastida
- kinetosome
- King, Charles Glen
- king crab
- kingdom
- kingfish
- King George’s War (1744–48)
- Kingitanga
- Kingman, Sir John Frank Charles (1939)
- Kingman, Sir John Frank Charles (1939– )
- King, Martin Luther (1929–68)
- King model
- King Philip’s War (1675–76)
- Kingsford Smith, Sir Charles (1897–1935)
- King, William Lyon Mackenzie (1874–1950)
- King William’s War (1689–97)
- kinin
- kink band
- kinked demand curve
- Kinnock, Neil Gordon, Baron Kinnock of Bedwellty (1942–)
- kinocilium
- kinomere
- kin selection