A statistic having a multivariate distribution which is the analogue of the univariate t-distribution. The statistic was introduced in 1931 by Hotelling. A sample of size n is drawn from a multivariate normal distribution with mean vector μ and variance–covariance matrix estimated as S. If the column vector of sample means is denoted by x̆ the statistic T² is given by 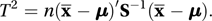In the case of p variables, {(n−p)T²}/{(n−1)p} has an F-distribution with p and (n−p) degrees of freedom. See also Mahalanobis D².