Any statistic of the form
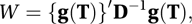 where T is an estimator of a vector parameter θ, g is some vector-valued function, and D is an estimator of the variance–covariance matrix of the vector {g(T) − g(θ)}. The statistics are used to test the null hypothesis that g(θ)=0, where 0 is a vector with all entries equal to 0. If T is the maximum likelihood estimator then W has an approximate chi-squared distribution with p degrees of freedom (where p is the number of elements in θ).
where T is an estimator of a vector parameter θ, g is some vector-valued function, and D is an estimator of the variance–covariance matrix of the vector {g(T) − g(θ)}. The statistics are used to test the null hypothesis that g(θ)=0, where 0 is a vector with all entries equal to 0. If T is the maximum likelihood estimator then W has an approximate chi-squared distribution with p degrees of freedom (where p is the number of elements in θ).
- event history analysis
- event horizon
- Event Horizon Telescope
- event input
- event manager
- event stratigraphy
- event stratinomy
- event tree analysis
- event-triggered email
- eventually
- even–even nucleus
- even–odd nucleus
- Everest, Mount
- Everett III, Hugh
- evergreen
- Evershed effect
- Evesham, Battle of (4 August 1265)
- evidence
- evidentialism
- evil genie
- evil, problem of
- evil twin
- EVN
- evocation
- evo-devo