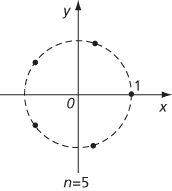
A complex number z such that zn = 1. The n distinct nth roots of unity are ei2kπ/n(k = 0, 1,…, n−1), or
They are represented in the complex plane by points that lie on the unit circle and are vertices of a regular n-sided polygon. The nth roots of unity for n = 5 and n = 6 are shown in the figure. The nth roots of unity always include the real number 1 and also include the real number−1 if n is even. The non-real nth roots of unity form pairs of conjugates.

- scaling analysis
- scaling function
- scaling law
- scaling tube
- scallop
- scan
- scandent
- Scandinavian ice sheet
- Scandinavian Journal of Statistics
- scandium
- Scanian War (1676–78)
- scan-line
- Scanlon, Thomas (1940– )
- scanned image
- scanner
- scanning
- scanning Auger microprobe
- scanning electron microscope
- scanning image absorption spectrometer for atmospheric cartography
- scanning laser
- scanning probe microscopy
- scanning-probe microscopy
- scanning-transmission electron microscope
- scanning tunnelling microscope
- scanning tunnelling microscopy