A measure of location that is not as sensitive as the mean to outlier values. With observations x₁, x₂,…, x_n, the sample mean can be characterized as the value of θ that minimizes , where g(u)=u². The sample median can be characterized in a similar way, though now g(u)=|u|.

M-estimates can be characterized in this same way, but the functional forms for g are chosen to be less sensitive to outlier values. One frequently used alternative is the Huber function: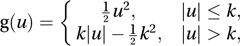where k is a tuning constant (often set equal to twice the median absolute deviation).

A second alternative is the biweight function: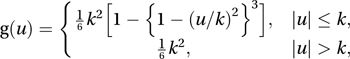where k is again a tuning constant and is here often set equal to seven times the median absolute deviation. See also L-estimate.