A non-parametric test of the null hypothesis that samples have been drawn from populations with a common distribution, with the alternative being that the distributions have the same mean (or median) but different scales (and thus different variances).

The test statistic (see hypothesis test) of the 1953 Rosenbaum test is the number of observations in one sample that exceed (or are less than) all the observations from the second sample.

The Mood dispersion test, introduced by Mood in 1954, is suitable for symmetric populations. The test is based on replacing the original values with their ranks in the combined sample. Suppose that the samples have sizes m and n, with m+n=N. The test statistic is M, given by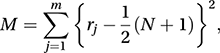where r_j is the overall rank in the combined sample of the jth observation in the sample of size m. If m and n are reasonably large and there are no tied ranks, then the transformed statistic z is an observation from an approximate standard normal distribution, where

The Barton–David test assumes a symmetric distribution, as does the Levene test, suggested by Levene in 1960 for comparing several populations. Let the kth observation in the jth of m samples be denoted by y_jk and let the mean of the n_j observations in this sample be denoted by y¯_j. Define z_jk by

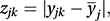
with the mean of the z-values in the jth sample being denoted by z¯_j and the overall mean of the n(=∑_jn_j) z-values being z¯. The Levene test statistic is W given by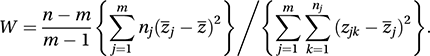If the null hypothesis of equality of variance is correct, then the distribution of W is approximately an F-distribution with (m−1) and (n−m) degrees of freedom.

A drawback of the Levene test is that the sample means may be affected by outliers. The Brown–Forsythe test, suggested in 1974, avoids this problem by working with z′_jk instead of z_jk, where

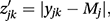
and M_j is the median of the jth sample.

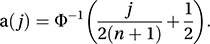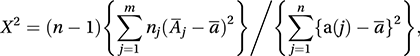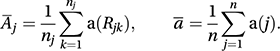A related test is the Fligner–Killeen test, suggested in 1976. Let R_jk be the rank of z′_jk amongst the n ordered z′_jk-values and define a(j) by:The test statistic, X², is given by:whereUnder the null hypothesis of equality of variances, X² is an observation from a chi-squared distribution with (m−1) degrees of freedom.

The Siegel–Tukey test introduced by Siegel and Tukey in 1960 uses alternatives to ranks that reflect the spread of the data. Denoting the N ordered values in the combined sample by x₍₁₎, x₍₂₎,…, x_(N−1), x_(N), these replacement values are determined as follows:

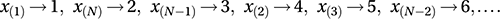
The test statistic is the sum of these values corresponding to the observations in the smaller sample.

Similarly motivated, the Ansari–Bradley test introduced by Ansari and Bradley in 1962 uses replacement values given by

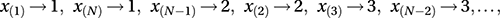
while the 1962 Klotz test is based on the replacement of the value of the rth largest of the N observations bySpecial tables (or exact calculation of tail probabilities) are required for most of these tests. See also test for equality of variance.