Sine is not a one-to-one function, and so not invertible, but ‘inverse sine’ can be defined by choosing a set of principal values. Thus, if y = sin−1 x, then x = sin y, but the converse need not hold. For each x in the interval [−1,1] there is a unique y in the interval [−π/2, π/2] such that x = sin y and this value of y is defined as y = sin−1 x.
Similarly, for real x, y = tan−1 x if x = tan y, and the unique value y is taken from the interval (−π/2, π/2). Also, for x in the interval [−1,1], y = cos−1 x if x = cos y and the unique value y is taken from the interval [0, π].
The notation arcsin, arctan, and arccos for sin−1, tan−1, and cos−1 is also used. Their derivatives are:
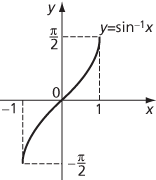
Graph of inverse sine
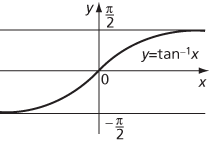
Graph of inverse tangent
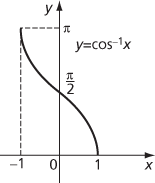
Graph of inverse cosine
- systems programming
- systems software
- systems theory
- systems tract
- system tables
- system temperature
- system testing
- System V
- systole
- systolic array
- Système International d’Unités
- Système Probatoire d’Observation de la Terre
- syzygy
- SZ
- Szemerédi’s theorem
- Szent-Györgyi, Albert von
- Szilard, Leo
- Szilard–Chalmers effect
- São Tomé and Príncipe
- Sèvres, Treaty of (1920)
- sövite
- Sālote Tupou III
- T
- t
- T2