A diagram in which the number of protons in a nucleus is plotted against the number of neutrons. This chart, named after the Italian-American physicist Emilio Segrè (1905–89), enables the narrow range of stable nuclei to be seen clearly and also illustrates that for more than twenty protons more neutrons than protons are required for stable nuclei.

The stability of nuclei can be understood qualitatively on the basis of the nature of the strong interaction (see fundamental interactions) and the competition between this attractive force and the repulsive electrical force. The strong interaction is independent of electric charge, i.e. at any given separation the strong force between two neutrons is the same as that between two protons or between a proton or a neutron. Therefore, in the absence of the electrical repulsion between protons, the most stable nuclei would be those having equal numbers of neutrons and protons. The electrical repulsion shifts the balance to favour a greater number of neutrons, but a nucleus with too many neutrons is unstable, because not enough of them are paired with protons.

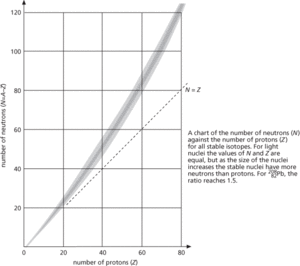
Segrè chart.

As the number of nucleons increases, the total energy of the electrical interaction increases faster than that of the nuclear interaction. The (positive, repulsive) electric potential energy of the nucleus increases approximately as Z², while the (negative, attractive) nuclear potential energy increases approximately as N+Z with corrections for pairing effects. For large N+Z values, the electrical potential energy per nucleon grows much faster than the nuclear potential energy per nucleon, until a point is reached where the formation of a stable nucleus is impossible. The competition between the electric and nuclear forces therefore accounts for the increase with Z of the neutron–proton ratio in stable nuclei as well as the existence of maximum values for N+Z for stability. See binding energy; decay; dripline; liquid-drop model.