The sample mean (or, simply, ‘the mean’) of a set of n items of data x₁, x₂,…, x_n is , which is the arithmetic average of the numbers x₁, x₂,…, x_n. The mean is usually denoted by placing a bar over the symbol for the variable being measured. If the variable is x the mean is denoted by . If the data constitute a sample from a population, then the sample mean is an unbiased estimate of the population mean.

For example, the numbers of eruptions of the Old Faithful geyser during the first eight days of August 1978 were 13, 13, 13, 14, 14, 14, 13, and 13. The mean is

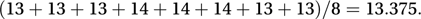
If the data are collected in frequency form so that values x₁, x₂,…, x_n are obtained with frequencies f₁, f₂,…, f_n the mean isFor example, with the eruption data there are just two values, x₁=13 and x₂=14. Their respective frequencies are f₁=5 and f₂=3, so the mean is {(5×13)+(3×14)}/(3+5)=13.375.

If the data are grouped into classes with mid-values x₁, x₂,…, x_c and corresponding class frequencies f₁, f₂,…, f_c, an approximate value for the mean of the original data is the grouped mean

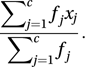
The mean can be interpreted as the centre of gravity, or centre of mass, of a system of particles of masses f₁, f₂,…,f_n at points x₁, x₁,…, x_n.

Sample mean. The data are the numbers of eruptions of the Old Faithful geyser during the first eight days of August 1978. The sample mean is seen to be the balance point of the observations.