1. The logical connective combining two statements or formulas P and Q in such a way that the outcome is true if both P and Q are true or if both are false, as shown in the table. P and Q are said to be equivalent. The connective can be read as ‘if and only if’ or ‘iff’, and is usually denoted by one of the following symbols:
See also exclusive-NOR gate, propositional calculus.2. A relationship between objects that are operationally or structurally indistinguishable, e.g. in combinational circuits, graphs, or grammars. Equivalence is less strong than identity or equality but much more useful in practice. See also machine equivalence
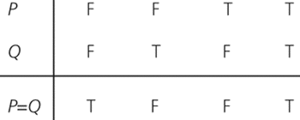
Equivalence. Truth table
.
A problem of non-uniqueness in the interpretation of electrical soundings, when identical trough- or peak-shaped apparent resistivity graphs for a three-layer case can be synthesized using different models for the middle layer. Identical peak-shaped graphs can be generated if the transverse resistance, Rt, remains a constant equal to hρ, where h is the thickness of the layer and ρ its true resistivity. Identical trough-shaped graphs can be generated if the longitudinal conductance, Gx, remains a constant equal to h/ρ. Such problems can be resolved if access is available to borehole geologic control and the range of h or ρ can be deduced.
- lucida
- Lucid, Shannon Matilda Wells (1943– )
- luciferase
- luciferin
- lucinoid
- Lucknow, Siege of (1857–58)
- Lucretius (99/94–55/51)
- Luddite
- Topa Inca
- topaz
- top carnivore
- top-down development
- top-down hierarchical design
- top-down nanofabrication
- top-down parsing
- tope
- TOPEX/Poseidon
- topic drift
- topic-neutral
- toplap
- top-level domain
- topocentric
- topocentric coordinates
- top of atmosphere
- topographic correction