For two random variables X and Y, this is the difference between the expected value of their product and the product of their separate expected values. It is denoted by Cov (X,Y):
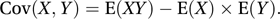
If X and Y are independent then Cov(X, Y)=0. However, if Cov(X, Y)=0 then X and Y may not be independent. A useful result is
Var(aX+bY)=a2Var(X)+2ab Cov(X, Y)+b2Var(Y), where Var denotes variance, and a and b are constants. The term 'covariance' was used by Sir Ronald Fisher in 1930. See also population correlation coefficient.
- latent learning
- latent period
- latent root
- latent variable
- latent vector
- latent virus
- lateral
- lateral accretion
- lateral accretion deposit
- lateral dispersion
- lateral erosion
- lateral gene transfer
- lateral inversion
- lateralization
- lateral-line system
- lateral moraine
- lateral root
- lateral thinking
- lateral velocity
- Lateran Council
- Lateran Treaties (11 February 1929)
- laterite
- laterolog sonde
- late-type galaxy
- late-type star